
 | ||||||
| Exponentially Decreasing Dose Rate Exposure Radiation Delivery |
All radioisotopes are unstable, and the intensity of the radiation emitted from a fixed quantity of a material decreases exponentially with time. Mathematically, the activity of a quantity of some radioactive material is given by A(t) = A(0)exp(-lt) where A(t) is the activity at time t and A(0) is the initial activity (time t = 0). The parameter l is a decay constant that characterizes the rate at which unstable atoms disintegrate (decay) and emit radiation. | Figure 1. Exponentially decreasing dose rate exposure scenario (click on figure to enlarge)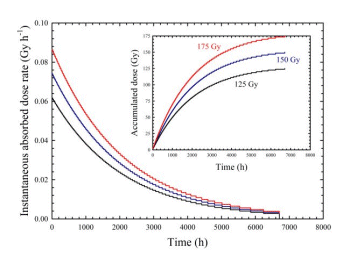 |
Definition: In the International System of Units (SI), the activity of a sample of radioactive material is specified in units called the becquerel (Bq). A Bq denotes one nuclear transformation (disintegration) per second. The Curie (Ci) is an other common (but non-SI) unit of activity. One curie equals 3.7 x 1010 Bq. The rate of radioactive decay is specific to the radioisotope. Often the decay rate is specified in terms of an alternate parameter called the half-life. The half-life T of a radioisotope is the amount of time required for half of the initial number of atoms to disintegrate, i.e., l = ln(2)/T ~ 0.693/T. Figure 1 shows examples of the instantaneous dose rate that may be experienced by cells near a 125I brachytherapy seed. The half-life for selected radioisotopes of interest in radiation therapy, nuclear medicine, and radiation protection is available elsewhere in the Virtual Cell manual (see the Table of half-lives for selected radioisotopes). | |
| School of Health Sciences Purdue University Disclaimer | Last updated: 10 June, 2011 |