CROSSOVER SCALING BETWEEN DYNAMIC UNIVERSALITY CLASSES.

Is There a z-Theorem for Dynamic Critical Exponents ?,
Marcel den Nijs, in the Proceedings of the 4th CTP Workshop on Statistical Physics: Dynamics of fluctuationg
Interfaces and Related Phenomena eds. D.Kim, H.Park, and B.Kahng, pages 272-292 (World Scientific, 1997).

The Crossover from Isotropic to Directed Percolation in 2 Dimensions,
Per Frojdh and Marcel den Nijs, Phys. Rev. Lett. 78, 1850 (1997).

Crossover Scaling Functions in One Dimensional Dynamic Growth Models,
John Neergaard and Marcel den Nijs, Phys.Rev.Lett. 74 730 (1995).

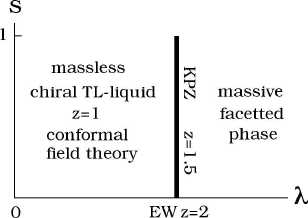
crossover scaling z-theorem

Crossover scaling between dynamic universality classes implies a link between the dynamic exponents of the two and the crossover exponents at the unstable process. I named this the z-theorem .
This property is especially useful when the crossover field is a a redundant stress tensor type operator for the stable process and the unstable one has known scaling properties, such that its dynamic exponent and the crossover exponent are known exactly.
An example is the crossover between Edwards-Wilkinson and KPZ type growth. In 1D, the KPZ dynamic exponent is therefore simply equal to z(KPZ)=z(EW)-y(cr)=2-0.5=1.5. In higher dimensions this does not work, because EW growth becomes stable and an intermediate unstable fixed point separates it from KPZ growth.

The edge of conformal field theory

D dimensional dynamic processes that can be formulated as Master equations are intrinsically related to time evolution operators in quantum field theory and transfer matrices of D+1 equilibrium statistical mechanics.
One example of this equivalence is the exact mapping between 1D KPZ growth and facet-ridge end-points in 2D equilibrium crystal shapes .

The scaling properties of 2D equilibrium critical phenomena have been largely understood nowadays in terms of conformal field theory and so-called Coulomb gas type representations (topological excitations in the Gaussian massless free scalar field theory). Those critical points however are typically not stochastic and have "dynamic" exponent z=1 because of rotational invariance in space (time in the dynamic process representation corresponds to the second spatial coordinate in the 2D equilibrium system).
Analytical progress in understanding dynamic processes beyond simulations and phenomelogical scaling theory, requires most likely an enhancement of conformal field theory to anisotropic scaling phenomena.
This is not a completely hopeless endeavor, because it turns out that in the world of non-unitary time evolution operators the stochastic ones appear to be located always at the edge of conformal field theory. I am not aware of any counter example.
The first figure to the right illustrates this for KPZ growth in the BCSOS model.
Another exampleis the crossover of isotropic (conventional) percolation (IP) to directed percolation (DP). The latter is the generic universality class for epidemic processes like forest fires and also surface catalysis.
In IP all bonds in the space time lattice are independently present or absent with probability p. In DP only bonds are allowed that connect to active sites at the previous instant in time. This means that the "spontaneous creation processes", labeled as c in the figure are frozen out.
We studied a the crossover between IP and DP by assigning a fugacity to the c process and thus freezing it out. The c type crossover exponent at IP is irrelevant, implying that systems with reduced c<1 belong to the IP universality class. DP at c=0 turns out to be unstable. This illustrates that again anisotropic scaling type dynamic process lies at the edge of conformal field theory. Moreover, unfortunately, the z-theorem does not provide useful information in this case since the unstable process (DP) is the non-trivial one.

spin-half quantum chain phase diagram

percolation cluster

home page of Marcel den Nijs