In this Monte Carlo study of the size of the weighted likelihood bootstrap, random samples were generated from a unit normal distribution with true mean set to values ranging from zero to .12. Simple bounds were placed on the estimate of the mean restricting it to the [0,20] interval. Confidence limits were computed by the weighted likelihood bootstrap and by inversion of the Wald statistic. For the weighted likelihood bootstrap, a uniform prior was imposed for the interval [0,20].
For this study, the re-sample sizes were set to 100. This increases
the magnitude of the stody by 100 times over that required for the
study reported in section 3.1. The results
for N = 500 and N = 800 are presented in Figure 5. The results for
the inverse Wald follow the pattern established in section 3.1:
the observed size is one half the theoretical size up to 
from the constraint boundary for N = 500, and .0693 for N = 800.
At that point the correct size is restored.
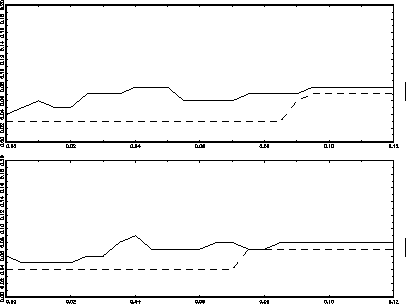
Figure 5: Size for Weighted Likelihood Bootstrap 95% Confidence Intervals, N = 500, 800
The size of the confidence limits for the weighted likelihood bootstrap do not appear to be influenced by the constraint boundary which is what we are expecting. The curve is somewhat irregular, however, and it may be the case that re-sample sizes greater than 100 are required for some stability of size. A re-sample size of 500 is reasonable for a single analysis, but would put a Monte Carlo study out of practical range.