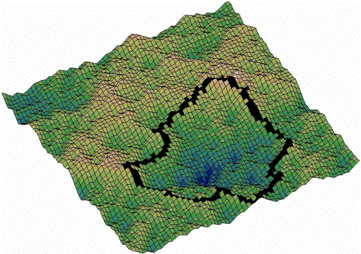
Growing surfaces are generically rough. Therefore the question whether surface reconstruction order can exist (in the rigorous thermodynamic sense of the word) must be addressed in the context of reconstructed rough phases.

The 2D so-called RSOS model with negative step energies is a compact model to couple an Ising field to surface roughness. In equilibrium it has an Ising transition inside the rough phase, into a checkerboard reconstructed rough phase. When the deposition rate exceeds the evaporation rate the same model describes KPZ growth (Kim-Kosterlitz model).

We find a peak in the susceptibility, which shifts with system size. Below it, power law tails in the time series histogram of the staggered magnetization imply the presence of quasi-critical reconstruction fluctuations

Ising type domain walls are being nucleated in valleys of the rough growing surface and are driven up-wards until they become trapped on the ridge lines of the surface.

There they remain trapped and slaved to the KPZ critical fluctuations. This is the origin of the quasi-critical fluctuations. At large length scales, new nucleation events dominate the annihilation of such loops, and imply that the reconstruction order only exists below a characteristic length scale.