Proton Single Particle Energy Shifts due to Coulomb Correlations
Aurel Bulgac ![]() and Vasily R. Shaginyan
and Vasily R. Shaginyan ![]()
![]() Department of Physics, University of Washington,
Seattle, WA 98195, USA
Department of Physics, University of Washington,
Seattle, WA 98195, USA
![]() Petersburg Nuclear Physics Institute, 188350 Gatchina,
RUSSIA
Petersburg Nuclear Physics Institute, 188350 Gatchina,
RUSSIA
We show that the interplay between the Coulomb interaction and the strong interaction, which is enhanced in the nuclear surface, leads to a significant upward shift of the proton single-particle levels. This shift affects the position of the calculated proton drip line, a shift towards decreasing Z by several units. The same mechanism is responsible for significant corrections to the mass difference of the mirror nuclei (Okamoto-Nolen-Schiffer anomaly) and to the effective proton mass.
PACS: 21.10.Sf Coulomb energies -- 21.10.Dr Binding energies -- 21.10.-k Nuclear energy levels
The main part of the Coulomb energy in nuclei is given by the Hartree
contribution and to a reasonable accuracy this can be computed as the
energy of a uniformly charged sphere and is thus proportional to
![]() . There are a number of corrections, some of them rather
subtle, arising from the interplay between the Coulomb and nuclear
forces. The Okamoto-Nolen-Schiffer anomaly in the binding energy of
mirror nuclei [1] is a case in point. In Ref. [2] we have
shown that a specific many-body mechanism (described briefly below)
leads to an enhancement of the Coulomb energy in the nuclear surface
region and in this way one can account for the major part of this
anomaly. This effect results in a systematic contribution to the
nuclear binding energy, which scales as
. There are a number of corrections, some of them rather
subtle, arising from the interplay between the Coulomb and nuclear
forces. The Okamoto-Nolen-Schiffer anomaly in the binding energy of
mirror nuclei [1] is a case in point. In Ref. [2] we have
shown that a specific many-body mechanism (described briefly below)
leads to an enhancement of the Coulomb energy in the nuclear surface
region and in this way one can account for the major part of this
anomaly. This effect results in a systematic contribution to the
nuclear binding energy, which scales as ![]() . We are
going to demonstrate that the mechanism should be taken into
account when calculating a number of nuclear properties. In this
Letter we study the role of this new many-body effect on the
single-particle proton energy levels, on the location of the proton
drip line and on the proton effective mass.
. We are
going to demonstrate that the mechanism should be taken into
account when calculating a number of nuclear properties. In this
Letter we study the role of this new many-body effect on the
single-particle proton energy levels, on the location of the proton
drip line and on the proton effective mass.
We shall operate within the density functional theory
[3, 4, 5, 6]. The ground state energy of a nucleus E is
given by a sum of two functionals (in the absence of
pairing correlations):
![]()
where the symmetric part ![]() is due to the (strong)
isospin conserving nuclear forces, while
is due to the (strong)
isospin conserving nuclear forces, while ![]() is due to the
(weak) Coulomb interaction. We shall neglect in our analysis several
easy to include terms: the trivial contribution in the kinetic energy,
arising from proton-neutron mass difference, the contribution of the
Charge Symmetry Breaking (CSB) forces [7]. For the sake of
simplicity of the presentation, w e shall not display explicitly the
spin degrees of freedom and the contribution arising from the
spin-orbit interaction, even though we have included them in the
actual calculations. The inclusion of all these terms does not change
our conclusions, it would merely lead to some rather small higher
order corrections. The proton and neutron densities are defined as
usual
is due to the
(weak) Coulomb interaction. We shall neglect in our analysis several
easy to include terms: the trivial contribution in the kinetic energy,
arising from proton-neutron mass difference, the contribution of the
Charge Symmetry Breaking (CSB) forces [7]. For the sake of
simplicity of the presentation, w e shall not display explicitly the
spin degrees of freedom and the contribution arising from the
spin-orbit interaction, even though we have included them in the
actual calculations. The inclusion of all these terms does not change
our conclusions, it would merely lead to some rather small higher
order corrections. The proton and neutron densities are defined as
usual
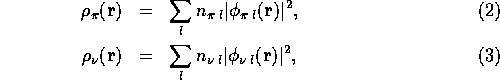
where ![]() and
and ![]() are
proton and neutron quasiparticle occupation numbers and
single-particle wave functions respectively. The well known Skyrme
functional, see e.g. Refs. [8, 9], can be considered as one
possible realization of
are
proton and neutron quasiparticle occupation numbers and
single-particle wave functions respectively. The well known Skyrme
functional, see e.g. Refs. [8, 9], can be considered as one
possible realization of ![]() . The Coulomb energy functional is given
by:
. The Coulomb energy functional is given
by:
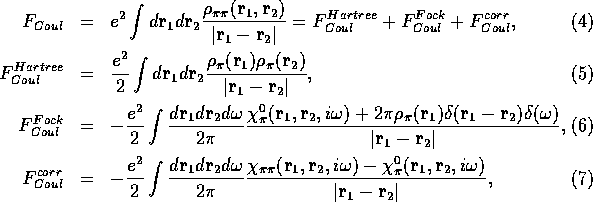
with ![]() being the exact two-proton
density distribution function.
being the exact two-proton
density distribution function. ![]() and
and ![]() are the
full and free proton linear response functions respectively, evaluated
at the imaginary frequency
are the
full and free proton linear response functions respectively, evaluated
at the imaginary frequency ![]() . Eqs. (5) and
(6) represent the Hartree and Fock contributions to the
Coulomb energy. The exchange term is written here in a somewhat
unusual way [2], through the linear response function of the
noninteracting protons,
. Eqs. (5) and
(6) represent the Hartree and Fock contributions to the
Coulomb energy. The exchange term is written here in a somewhat
unusual way [2], through the linear response function of the
noninteracting protons, ![]() , since upon integrating along the
real axis of the complex
, since upon integrating along the
real axis of the complex ![]() plane one has
plane one has
![]()
where ![]() is the proton single-particle
density matrix [10]. In
Eqs. (6,7) we have performed a Wick rotation
in order to evaluate the integral along the imaginary axis. Upon
taking into account the (strong) residual interaction, the linear free
response function
is the proton single-particle
density matrix [10]. In
Eqs. (6,7) we have performed a Wick rotation
in order to evaluate the integral along the imaginary axis. Upon
taking into account the (strong) residual interaction, the linear free
response function ![]() should be replaced with the full
response function
should be replaced with the full
response function ![]() and one thus readily obtains the
expression for the Coulomb correlation energy (7). By
considering all three contributions
(5,6,7) we therefore account
for all diagrams in first order in
and one thus readily obtains the
expression for the Coulomb correlation energy (7). By
considering all three contributions
(5,6,7) we therefore account
for all diagrams in first order in ![]() , in the (weak) Coulomb
interaction. The (strong) nuclear interaction is treated to all
orders. We are going to concentrate on a study of the contribution to
the single particle levels and the effective mass due to
Eq. (7). We remark that there are no problems with double
counting, rearrangement energy, core-polarization correction, etc. in
the density functional formalism.
, in the (weak) Coulomb
interaction. The (strong) nuclear interaction is treated to all
orders. We are going to concentrate on a study of the contribution to
the single particle levels and the effective mass due to
Eq. (7). We remark that there are no problems with double
counting, rearrangement energy, core-polarization correction, etc. in
the density functional formalism.
By expressing ![]() through the linear response function
we can easily calculate a number of functional derivatives needed
below. At the same time one can clearly disentangle the contribution
of various modes to this part of the energy density functional as well
[4]. In Ref. [2] we have shown that the main contribution
to
through the linear response function
we can easily calculate a number of functional derivatives needed
below. At the same time one can clearly disentangle the contribution
of various modes to this part of the energy density functional as well
[4]. In Ref. [2] we have shown that the main contribution
to ![]() comes from the surface collective isoscalar
excitations. In the isoscalar channel the particle-hole residual
interaction has a strong density dependence, changing from a
relatively weak one inside nuclei to a strong attractive one in the
surface region [11]. Because of the attractive character of
the isoscalar residual particle-hole interaction, the nuclear surface
(where the matter density is low) is very close to instability
[2]. Low density homogeneous nuclear matter is manifestly
unstable and this shows itself in the fact that the linear response
function has a pole for imaginary values of
comes from the surface collective isoscalar
excitations. In the isoscalar channel the particle-hole residual
interaction has a strong density dependence, changing from a
relatively weak one inside nuclei to a strong attractive one in the
surface region [11]. Because of the attractive character of
the isoscalar residual particle-hole interaction, the nuclear surface
(where the matter density is low) is very close to instability
[2]. Low density homogeneous nuclear matter is manifestly
unstable and this shows itself in the fact that the linear response
function has a pole for imaginary values of ![]() [2]. In
finite nuclei and semi-infinite nuclear matter this singularity is
smoothed out and becomes a prominent peak of the response function at
low frequencies in the surface region. This proximity of the nuclear
surface to instability is the reason why the contribution of the
(weak) Coulomb exchange interaction is strongly enhanced and the
correlation Coulomb ``correction'' to the mass formula has as a result
a predominantly surface character, i.e
[2]. In
finite nuclei and semi-infinite nuclear matter this singularity is
smoothed out and becomes a prominent peak of the response function at
low frequencies in the surface region. This proximity of the nuclear
surface to instability is the reason why the contribution of the
(weak) Coulomb exchange interaction is strongly enhanced and the
correlation Coulomb ``correction'' to the mass formula has as a result
a predominantly surface character, i.e ![]() .
.
Let us turn now to the calculation of the proton single-particle
energy corrections due to the presence of ![]() in the
energy functional. Using Landau's variational equation [12]
in the
energy functional. Using Landau's variational equation [12]
![]()
one obtains for the proton single-particle energy shift
![]() the following expression
the following expression
![]()
The variational derivative ![]() has the simple functional form,
has the simple functional form,
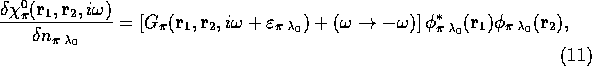
with ![]() being the single-particle
proton propagator in the selfconsistent nuclear potential. The linear
response function
being the single-particle
proton propagator in the selfconsistent nuclear potential. The linear
response function ![]() is obtained by solving the usual
matrix functional equation (Landau zero sound or RPA)
is obtained by solving the usual
matrix functional equation (Landau zero sound or RPA)
![]()
where ![]() is the irreducible (strong) particle-hole interaction
and i,j and k stand for the isospin variables,
is the irreducible (strong) particle-hole interaction
and i,j and k stand for the isospin variables, ![]() and
and ![]() for protons and neutrons respectively. From this equation one derives
the following equation for
for protons and neutrons respectively. From this equation one derives
the following equation for ![]()
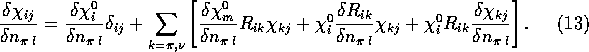
In Ref. [2] we have used a simple
separable model for the residual interaction ![]()
![]()
where ![]() is the proton/neutron single-particle potential in
a spherical nucleus and
is the proton/neutron single-particle potential in
a spherical nucleus and ![]() is a Dirac
function in angle variables.
is a Dirac
function in angle variables. ![]() is chosen so that the dipole
linear response has a pole at
is chosen so that the dipole
linear response has a pole at ![]() , corresponding to the
spurious mode. This type of residual interaction has been studied
extensively [8, 13, 14] and it leads to a satisfactory description
of nuclear collective modes. We have used the same nonselfconsistent
approach described in detail in Ref. [2] in order to estimate
the magnitude of the correction
, corresponding to the
spurious mode. This type of residual interaction has been studied
extensively [8, 13, 14] and it leads to a satisfactory description
of nuclear collective modes. We have used the same nonselfconsistent
approach described in detail in Ref. [2] in order to estimate
the magnitude of the correction ![]() . For
various single-particle proton levels around the Fermi level and the
proton threshold, the calculated shifts
. For
various single-particle proton levels around the Fermi level and the
proton threshold, the calculated shifts ![]() are in the interval 0.1-0.3 MeV in light (A=16) and medium
(A=40-48) nuclei. In performing these calculations in each nucleus
we have included collective modes with multipolarities up to
are in the interval 0.1-0.3 MeV in light (A=16) and medium
(A=40-48) nuclei. In performing these calculations in each nucleus
we have included collective modes with multipolarities up to ![]() , see Ref. [2]. The values of
, see Ref. [2]. The values of ![]() thus obtained are of the same magnitude as the
Okamoto-Nolen-Schiffer anomaly.
thus obtained are of the same magnitude as the
Okamoto-Nolen-Schiffer anomaly.
It is instructive to cross check these results using an independent approach. A new type of nuclear density functionals has been recently introduced in Refs. [5, 6], see also Ref. [9]. The main reason for seeking new functionals is to obtain a significantly more accurate reproduction of the nuclear properties (bindings energies and matter distribution of finite nuclei and infinite neutron and symmetric nuclear matter properties over a wide range of densities simultaneously) than one can achieve with the plethora of existing density functionals. A key ingredient was the introduction of the Coulomb correlation energy contribution, along the lines suggested earlier by us in Refs. [2]. However, while we have presented arguments for a significant surface contribution into the nuclear Coulomb correlation energy, Fayans [5] has chosen to parametrize the Coulomb correlation energy as a volume term:
![]()
Here ![]() fm
fm![]() ,
, ![]() and from a fit of the
masses and radii of 100 medium and heavy spherical nuclei Fayans
determined
and from a fit of the
masses and radii of 100 medium and heavy spherical nuclei Fayans
determined ![]() . The Coulomb correlation energy determined
by Fayans thus almost exactly cancels the Coulomb exchange energy
(which is formally given by the same formula with
. The Coulomb correlation energy determined
by Fayans thus almost exactly cancels the Coulomb exchange energy
(which is formally given by the same formula with ![]() and
and
![]() ), see also Ref. [9]. A quick estimate of this
contribution shows that
), see also Ref. [9]. A quick estimate of this
contribution shows that
![]()
which leads to a typical shift ![]() of the same order of magnitude as
estimated by us independently. An upward shift of this magnitude of
the last occupied proton level in a nucleus near the proton drip line
is equivalent to a shift of the proton drip line in the direction of
decreasing Z by a few units. A shift of this magnitude for a
neutron level would be equivalent to changing the mass number by up to
5 units, see for example [15]. A similar shift due to the
Coulomb interaction arises for neutron levels as well, but we shall
not discuss this mechanism here.
of the same order of magnitude as
estimated by us independently. An upward shift of this magnitude of
the last occupied proton level in a nucleus near the proton drip line
is equivalent to a shift of the proton drip line in the direction of
decreasing Z by a few units. A shift of this magnitude for a
neutron level would be equivalent to changing the mass number by up to
5 units, see for example [15]. A similar shift due to the
Coulomb interaction arises for neutron levels as well, but we shall
not discuss this mechanism here.
One can show that there is another related effect due to the Coulomb
correlation energy, a noticeable renormalization of the proton
effective mass near the Fermi surface. In the case of homogeneous
nuclear matter the effective mass is defined as [12]
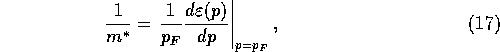
where ![]() is the Fermi momentum. Using Eq. (10) one obtains
that the effective mass renormalization
is the Fermi momentum. Using Eq. (10) one obtains
that the effective mass renormalization ![]() can be obtained
from the relation
can be obtained
from the relation
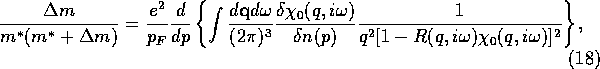
where ![]() is the proton effective mass computed in the absence of
the Coulomb correlation energy and n(p) is the quasiparticle
occupation number probability of the state with linear momentum
p. Using the following approximate formula (which becomes an
identity at the point where the compressibility is vanishing)
is the proton effective mass computed in the absence of
the Coulomb correlation energy and n(p) is the quasiparticle
occupation number probability of the state with linear momentum
p. Using the following approximate formula (which becomes an
identity at the point where the compressibility is vanishing)
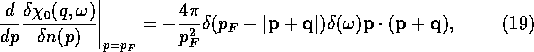
one can reduce the above expression for the effective mass shift to
![]()
where ![]() At the point where the compressibility
tends to zero, the denominator
At the point where the compressibility
tends to zero, the denominator ![]() vanishes
at
vanishes
at ![]() . We have shown in Ref. [2] that the nuclear
surface of finite nuclei is rather close to this regime. Since the
integrand in Eq. (20) is positive, the integral diverges and
the effective mass thus vanishes in an infinite homogeneous system.
In finite nuclei this divergence is smoothed out and the pole
singularity becomes a narrow surface peak [2]. The net effect is
that the proton effective mass becomes smaller than the neutron
effective mass, and therefore the proton level density is smaller than
the neutron level density in nuclei near the proton drip
line. B.A. Brown argues that a somewhat similar in medium effective
nucleon mass renormalization can account for the
Okamoto-Nolen-Schiffer anomaly [9]. An earlier QCD sum-rule
approach [16] substantiate such a claim. This effect, as well as
the contributions arising from CSB forces [7], lead mainly to
volume effects, while the many-body mechanism discussed by us leads
to a surface contribution. There is thus hope not only to generate in
the near future extremely accurate nuclear density functionals, but
also to be able to understand rather well the nature of various rather
subtle contributions. We anticipate as well that the study of the
Okamoto-Nolen-Schiffer anomaly within the framework of the density
functional approach will allow us to estimate the magnitude and the
character (volume/surface) of the CSB many-body effects.
. We have shown in Ref. [2] that the nuclear
surface of finite nuclei is rather close to this regime. Since the
integrand in Eq. (20) is positive, the integral diverges and
the effective mass thus vanishes in an infinite homogeneous system.
In finite nuclei this divergence is smoothed out and the pole
singularity becomes a narrow surface peak [2]. The net effect is
that the proton effective mass becomes smaller than the neutron
effective mass, and therefore the proton level density is smaller than
the neutron level density in nuclei near the proton drip
line. B.A. Brown argues that a somewhat similar in medium effective
nucleon mass renormalization can account for the
Okamoto-Nolen-Schiffer anomaly [9]. An earlier QCD sum-rule
approach [16] substantiate such a claim. This effect, as well as
the contributions arising from CSB forces [7], lead mainly to
volume effects, while the many-body mechanism discussed by us leads
to a surface contribution. There is thus hope not only to generate in
the near future extremely accurate nuclear density functionals, but
also to be able to understand rather well the nature of various rather
subtle contributions. We anticipate as well that the study of the
Okamoto-Nolen-Schiffer anomaly within the framework of the density
functional approach will allow us to estimate the magnitude and the
character (volume/surface) of the CSB many-body effects.